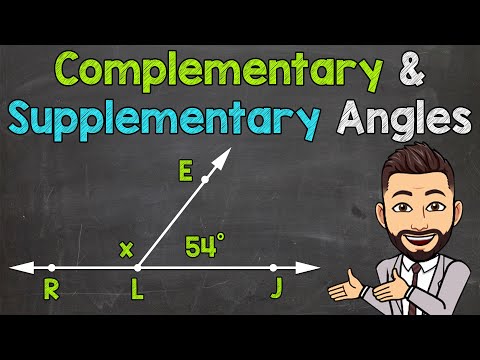
Complementary angles are those angles whose sum is equal to 90o. Thus, complementary angles are always acute angles. Hence, two acute angles can be complementary.
Q. What are complementary angles?
Two angles are called complementary when their measures add to 90 degrees. Two angles are called supplementary when their measures add up to 180 degrees.
Table of Contents
- Q. What are complementary angles?
- Q. What is the complementary angle of 90?
- Q. Can three angles be complementary?
- Q. Can 2 obtuse angles be supplementary?
- Q. Can 3 angles be supplementary?
- Q. How do you know if angles are congruent or supplementary?
- Q. How do you know when an angle is supplementary?
- Q. Can vertical angles be supplementary?
- Q. Are 2 vertical angles always supplementary?
- Q. What is the difference between vertical angles and supplementary angles?
- Q. Are vertical angles congruent or supplementary?
- Q. Are same side interior angles supplementary or congruent?
- Q. How do you prove angles are congruent?
- Q. What proves that vertical angles are congruent?
- Q. How do you prove two lines are parallel?
- Q. Can same side interior angles be congruent?
- Q. Are vertical angles SAS?
- Q. What is SAS ASA SSS AAS?
- Q. What is vertical angles Theorem?
- Q. What is a SAS triangle?
- Q. How do you prove SAS?
- Q. What is SAS formula?
- Q. What is SAS congruence rule?
Q. What is the complementary angle of 90?
Complementary angle of 90o is 0o and supplementary angle of 90o is 90o .
Q. Can three angles be complementary?
Three angles or more angles whose sum is equal to 90 degrees cannot also be called complementary angles. Complementary angles always have positive measures. It is composed of two acute angles measuring less than 90 degrees.
Q. Can 2 obtuse angles be supplementary?
Can 2 Obtuse Angles be Supplementary Angles? No, two obtuse angles cannot form a supplementary angle. By the definition of obtuse angles, the angles that measure greater than 90° are obtuse. If you add two obtuse angles, the sum will be greater than 180°.
Q. Can 3 angles be supplementary?
Notice the only sets that sum to 180° are the first, fifth, sixth and eighth pairs. Only those pairs are supplementary angles. The third set has three angles that sum to 180° ; three angles cannot be supplementary.
Q. How do you know if angles are congruent or supplementary?
A linear pair forms a straight angle which contains 180º, so you have 2 angles whose measures add to 180, which means they are supplementary. If two congruent angles form a linear pair, the angles are right angles. If two congruent angles add to 180º, each angle contains 90º, forming right angles.
Q. How do you know when an angle is supplementary?
Two Angles are Supplementary when they add up to 180 degrees. Notice that together they make a straight angle. But the angles don’t have to be together.
Q. Can vertical angles be supplementary?
Vertical angles are supplementary angles when the lines intersect perpendicularly. For example, ∠W and ∠ Y are vertical angles which are also supplementary angles.
Q. Are 2 vertical angles always supplementary?
Vertical angles are always congruent, or of equal measure. Both pairs of vertical angles (four angles altogether) always sum to a full angle (360°). Adjacent angles. In the figure above, an angle from each pair of vertical angles are adjacent angles and are supplementary (add to 180°).
Q. What is the difference between vertical angles and supplementary angles?
Supplementary angles are two angles with a sum of 180º. Vertical angles are two angles whose sides form two pairs of opposite rays. We can think of these as opposite angles formed by an X.
Q. Are vertical angles congruent or supplementary?
When two lines intersect they form two pairs of opposite angles, A + C and B + D. Another word for opposite angles are vertical angles. Vertical angles are always congruent, which means that they are equal. Adjacent angles are angles that come out of the same vertex.
Q. Are same side interior angles supplementary or congruent?
Same side interior angles are on the same side of the transversal. Same side interior angles are congruent when lines are parallel.
Q. How do you prove angles are congruent?
Two angles are congruent if they have the same measure. You already know that when two lines intersect the vertical angles formed are congruent.
Q. What proves that vertical angles are congruent?
So we will prove those congruent. Draw two intersecting lines similar to those above using a heavy marker on patty paper. (It is not important to match the angle) Fold the paper along a line that also goes through the point of intersection. Therefore, vertical angles are congruent.
Q. How do you prove two lines are parallel?
If two lines are cut by a transversal and the alternate exterior angles are equal, then the two lines are parallel. Angles can be equal or congruent; you can replace the word “equal” in both theorems with “congruent” without affecting the theorem. So if ∠B and ∠L are equal (or congruent), the lines are parallel.
Q. Can same side interior angles be congruent?
Q. Are vertical angles SAS?
SAS; two pairs of corresponding sides and their included vertical angles are congruent. SAS; two pairs of corresponding sides and their included right angle are congruent. SSS or SAS; three pairs of corresponding sides are congruent, or, two pairs of corresponding sides and their included vertical angles are congruent.
Q. What is SAS ASA SSS AAS?
SAS (side-angle-side) Two sides and the angle between them are congruent. ASA (angle-side-angle) Two angles and the side between them are congruent. AAS (angle-angle-side)
Q. What is vertical angles Theorem?
Theorem:Vertical angles are always congruent. In the figure, ∠1≅∠3 and ∠2≅∠4. Proof: ∠1and∠2 form a linear pair, so by the Supplement Postulate, they are supplementary.
Q. What is a SAS triangle?
SAS stands for “side, angle, side” and means that we have two triangles where we know two sides and the included angle are equal.
Q. How do you prove SAS?
Side-Angle-Side (SAS) Rule Side-Angle-Side is a rule used to prove whether a given set of triangles are congruent. The SAS rule states that: If two sides and the included angle of one triangle are equal to two sides and included angle of another triangle, then the triangles are congruent.
Q. What is SAS formula?
Using the SAS area formula, you can find the area of a triangle if you know the length of two sides of a triangle and included angle. Specifically, if the sides of the included angle are symbolized as b and c, and the included angle is called A, then: area of triangle ABC = (b*c*sin A) / 2.
Q. What is SAS congruence rule?
SAS (Side-Angle-Side) If any two sides and the angle included between the sides of one triangle are equivalent to the corresponding two sides and the angle between the sides of the second triangle, then the two triangles are said to be congruent by SAS rule.