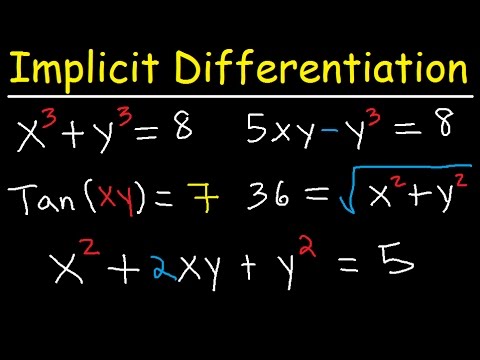
In implicit differentiation, we differentiate each side of an equation with two variables (usually x and y) by treating one of the variables as a function of the other. This calls for using the chain rule. Let’s differentiate x 2 + y 2 = 1 x^2+y^2=1 x2+y2=1x, squared, plus, y, squared, equals, 1 for example.
Q. What does explicit 5 points mean?
stated clearly
Table of Contents
- Q. What does explicit 5 points mean?
- Q. What is implicit function with example?
- Q. How do you differentiate an explicit function?
- Q. How do you differentiate implicitly XY?
- Q. What’s derivative mean?
- Q. How does chain rule work?
- Q. What does it mean for a function to have a limit?
- Q. What is limit definition of derivative?
- Q. What is the relationship between derivatives and limits?
- Q. How do you take the Antiderivative?
Q. What is implicit function with example?
In mathematics, an implicit equation is a relation of the form R(x1,…, xn) = 0, where R is a function of several variables (often a polynomial). For example, the equation x2 + y2 − 1 = 0 of the unit circle defines y as an implicit function of x if –1 ≤ x ≤ 1, and one restricts y to nonnegative values.
Q. How do you differentiate an explicit function?
Finding the derivative explicitly is a two-step process: (1) find y in terms of x, and (2) differentiate, which gives us dy/dx in terms of x. Finding the derivative implicitly is also two steps: (1) differentiate, and (2) solve for dy/dx. This method may leave us with dy/dx in terms of both x and y.
Q. How do you differentiate implicitly XY?
Implicit differentiation of (x-y)²=x+y-1.
Q. What’s derivative mean?
A derivative is a contract between two or more parties whose value is based on an agreed-upon underlying financial asset (like a security) or set of assets (like an index). Common underlying instruments include bonds, commodities, currencies, interest rates, market indexes, and stocks.
Q. How does chain rule work?
The chain rule states that the derivative of f(g(x)) is f'(g(x))⋅g'(x). In other words, it helps us differentiate *composite functions*. For example, sin(x²) is a composite function because it can be constructed as f(g(x)) for f(x)=sin(x) and g(x)=x².
Q. What does it mean for a function to have a limit?
A limit tells us the value that a function approaches as that function’s inputs get closer and closer to some number. The idea of a limit is the basis of all calculus.
Q. What is limit definition of derivative?
The derivative of function f at x=c is the limit of the slope of the secant line from x=c to x=c+h as h approaches 0. Symbolically, this is the limit of [f(c)-f(c+h)]/h as h→0. Created by Sal Khan.
Q. What is the relationship between derivatives and limits?
Since the derivative is defined as the limit which finds the slope of the tangent line to a function, the derivative of a function f at x is the instantaneous rate of change of the function at x.
Q. How do you take the Antiderivative?
To find an antiderivative for a function f, we can often reverse the process of differentiation. For example, if f = x4, then an antiderivative of f is F = x5, which can be found by reversing the power rule. Notice that not only is x5 an antiderivative of f, but so are x5 + 4, x5 + 6, etc.