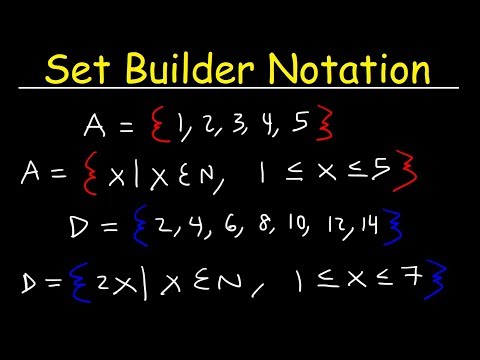
Describing sets

There are three main ways to identify a set:

• A written description,
• List or Roster method,
• Set builder Notation,

A set in mathematics is a collection of well defined and distinct objects, considered as an object in its own right. The most basic properties are that a set “has” elements, and that two sets are equal (one and the same) if and only if every element of one is an element of the other.

1. specifying a rule or a verbal description. For example, one can say “let A be the set of all odd integers”.
2. enclosing the list of members within curly brackets. For example, C={2,4,5} denotes a set of three numbers: 2, 4, and 5, and D={(2,4),(−1,5)} denotes a set of two pairs of numbers.

Bulusuchaitanya wrote: Find the greatest number that will divide 43, 91 and 183 so as to leave the same remainder in each case. Greatest number=HCF(91-43, 183-91, 183-43)=HCF(48, 92, 140)=4.

To find out HCF of three given numbers using division method, Step 1: Find out HCF of any two numbers. Step 2: Find out the HCF of the third number and the HCF obtained in step 1. Step 3: HCF obtained in step 2 will be the HCF of the three numbers.

Answer. Answer: The number will be HCF of 307 – 3 = 304 and 330 – 7 = 323.

Reminder 2. Therefore 110–2=108 AND 128–2=126 are fully divisible.

Hence the greatest number is 45.

We have to find the greatest number that will divide 2400 and 1810 and leave remainders 6 and 4 respectively. So, the required number will divide (2400 – 6) = 2394 and (1810 – 4) = 1806 completely with zero remainder. Therefore, the required number is the greatest common factor of 2394 and 1806.