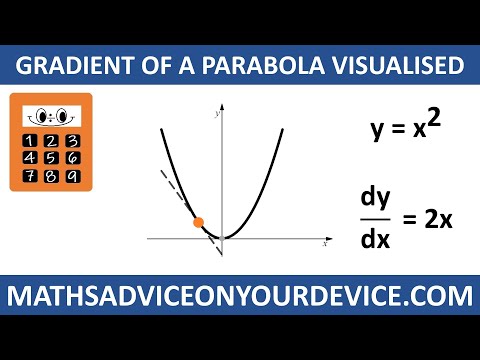
Q. How do you find the gradient of a parabola?
Method: Average Gradient
- Write the equation of the curve in the form y=….
- Calculate y1 by substituting x1 into the equation for the curve.
- Calculate y2 by substituting x2 into the equation for the curve.
- Calculate the average gradient using: y2-y1x2-x1.
Q. What is a 1 in 10 gradient in degrees?
Slopes vs. gradients vs. % grades
| Slope | ||
|---|---|---|
| Angle (degrees) | Gradient | Grade (%) |
| 9 | 1 | 15.8 |
| 10 | 1 | 17.6 |
| 11 | 1 | 19.4 |
Q. What is a 1 in 80 gradient?
A gradient of 1 in 80 is converted to a number instead of a ratio. 1 / 80 = 0.0125.
Q. What is a 1 in 60 gradient?
A gradient of 1:60 means that there will be 1 unit of fall for every 60 units of patio width. The patio is to be 4.2m wide, so if that distance (the run) is divided by 60, the result is the 1 unit of fall.
Q. What is a gradient percentage?
In cycling terms, “gradient” simply refers to the steepness of a section of road. A flat road is said to have a gradient of 0%, and a road with a higher gradient (e.g. 10%) is steeper than a road with a lower gradient (e.g. 5%).
Q. What is a 1 in 100 fall?
A 1:100 slope means that for every 100 metres along the ground, the slope height increases by 1 metre. A 1:0.5 slope means that for every 1 metre along the ground, the slope height increases by 0.5 metres.
Q. What’s a gradient?
The Gradient (also called Slope) of a straight line shows how steep a straight line is.
Q. Can you take the gradient of a vector?
No, gradient of a vector does not exist. Gradient is only defined for scaler quantities. Gradient converts a scaler quantity into a vector.
Q. What is the gradient of a triangle?
Finding the gradient choose any two points on the line. draw a right-angled triangle from one to the other, using the line as the hypotenuse. determine the height and width of the triangle. gradient = height ÷ width.
