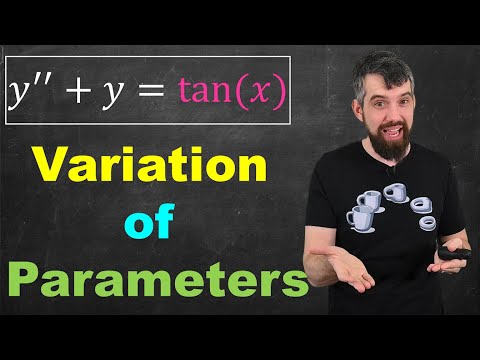
where p and q are constants and f(x) is a non-zero function of x. The complete solution to such an equation can be found by combining two types of solution: The general solution of the homogeneous equation d2ydx2 + pdydx + qy = 0.

: a method for solving a differential equation by first solving a simpler equation and then generalizing this solution properly so as to satisfy the original equation by treating the arbitrary constants not as constants but as variables.

Joseph Louis Lagrange

Let f be a differential equation with general solution F. A parameter of F is an arbitrary constant arising from the solving of a primitive during the course of obtaining the solution of f.

In mathematics, the Wronskian (or Wrońskian) is a determinant introduced by Józef Hoene-Wroński (1812) and named by Thomas Muir (1882, Chapter XVIII). It is used in the study of differential equations, where it can sometimes show linear independence in a set of solutions.

If f and g are two differentiable functions whose Wronskian is nonzero at any point, then they are linearly independent. If f and g are both solutions to the equation y + ay + by = 0 for some a and b, and if the Wronskian is zero at any point in the domain, then it is zero everywhere and f and g are dependent.

This is a system of two equations with two unknowns. The determinant of the corresponding matrix is the Wronskian. Hence, if the Wronskian is nonzero at some t0, only the trivial solution exists. Hence they are linearly independent.

A nontrivial solution is a solution that is not identically the zero function. Definition 13 Fundamental Set of Solutions. A set S of n linearly independent nontrivial solutions of the nth-order linear homogeneous equation (4.5) is called a fundamental set of solutions of the equation.

We have now found a test for determining whether a given set of vectors is linearly independent: A set of n vectors of length n is linearly independent if the matrix with these vectors as columns has a non-zero determinant. The set is of course dependent if the determinant is zero.

Cosine and Sine Functions are Linearly Independent.

If m > n then there are free variables, therefore the zero solution is not unique. Two vectors are linearly dependent if and only if they are parallel. Therefore v1,v2,v3 are linearly independent. Four vectors in R3 are always linearly dependent.

Solution: No, they cannot span all of R4. Any spanning set of R4 must contain at least 4 linearly independent vectors. Our set contains only 4 vectors, which are not linearly independent. The dimension of R3 is 3, so any set of 4 or more vectors must be linearly dependent.

: the property of a set (as of matrices or vectors) having no linear combination of all its elements equal to zero when coefficients are taken from a given set unless the coefficient of each element is zero.

In the end, the significance of linear independence is that you can uniquely represent members of the span of that set in terms of that set itself.

1 : made up of, relating to, or like a line : straight. 2 : involving a single dimension. linear.