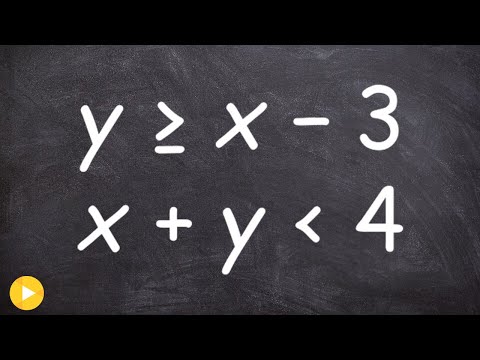Steps on Graphing Linear Inequalities

1. Step 1: Always start by isolating the variable y on the left side of the inequality.
2. Step 2: Change the inequality to equality symbol.
3. Step 3: Graph the boundary line from step 2 in the X Y − XY- XY−plane.
4. Step 4: The last step is to shade one side or region of the boundary line.

The technique for solving these systems is fairly simple. Here’s an example. “Solving” systems of linear inequalities means “graphing each individual inequality, and then finding the overlaps of the various solutions”. So I graph each inequality, and then find the overlapping portions of the solution regions.

The graph of an inequality in two variables is the set of points that represents all solutions to the inequality. A linear inequality divides the coordinate plane into two halves by a boundary line where one half represents the solutions of the inequality. The boundary line is dashed for > and < and solid for ≤ and ≥.

Linear inequalities with two variables have infinitely many ordered pair solutions, which can be graphed by shading in the appropriate half of a rectangular coordinate plane. To graph the solution set of an inequality with two variables, first graph the boundary with a dashed or solid line depending on the inequality.

There are three steps:

1. Rearrange the equation so “y” is on the left and everything else on the right.
2. Plot the “y=” line (make it a solid line for y≤ or y≥, and a dashed line for y< or y>)
3. Shade above the line for a “greater than” (y> or y≥) or below the line for a “less than” (y< or y≤).

In mathematics a linear inequality is an inequality which involves a linear function. A linear inequality contains one of the symbols of inequality:. It shows the data which is not equal in graph form. A linear inequality looks exactly like a linear equation, with the inequality sign replacing the equality sign.

There are three basic methods of graphing linear functions. The first is by plotting points and then drawing a line through the points. The second is by using the y-intercept and slope. The third is applying transformations to the identity function f(x)=x f ( x ) = x .

The standard form for linear equations in two variables is Ax+By=C. For example, 2x+3y=5 is a linear equation in standard form. When an equation is given in this form, it’s pretty easy to find both intercepts (x and y).

Answer Expert Verified eliminating systematic measurement errors caused by errors in the measuring instrument used to compare the quantity being measured with a standard.

Answer: The linear inequality in two variables is 2x – 3y ≤ 8. In other words, the answer is the letter C.

If a, b, and r are real numbers (and if a and b are not both equal to 0) then ax+by = r is called a linear equation in two variables. (The “two variables” are the x and the y.) The numbers a and b are called the coefficients of the equation ax+by = r. The number r is called the constant of the equation ax + by = r.

1 solution

Therefore, the solution set of a single linear inequality is always a half plane, so there are infinitely many solutions. So, for a linear inequality in two variables, there are infinitely many numbers of solutions.

Linear equations with two variables may appear in the form Ax + By = C, and the resulting graph is always a straight line. More often, the equation takes the form y = mx + b, where m is the slope of the line of the corresponding graph and b is its y-intercept, the point at which the line meets the y-axis.

The linear equations in one variable is an equation which is expressed in the form of ax+b = 0, where a and b are two integers, and x is a variable and has only one solution. For example, 2x+3=8 is a linear equation having a single variable in it.

Given the graph of a line, you can determine the equation in two ways, using slope-intercept form, y=mx+b y = m x + b , or point-slope form, y−y1= m(x−x1) y − y 1 = m ( x − x 1 ) . The slope and one point on the line is all that is needed to write the equation of a line.

A linear equation is an equation with two variables whose graph is a line. The graph of the linear equation is a set of points in the coordinate plane that all are solutions to the equation. When choosing your points try to include both positive and negative values as well as zero.

A line graph plots data in a single line over time. To describe the graph, follow it’s progress along the horizontal access and describe whether it goes down, up, or stays the same.

Why is it Called a Linear Equation? It is called a linear equation, because if you try to plot the graph of the given equation with variables x and y on a graph with axes as x and y, you would get a line as your result. Hence, it is called a linear equation.

Real life examples include:

• Calculating wages based on an hourly pay rate.
• Calculating medicine doses based on patients’ weights.
• Calculating the perimeters of squares.
• Hiring a car if a deposit is paid and there is an hourly charge.

A linear function has one independent variable and one dependent variable. The independent variable is x and the dependent variable is y. Step-by-step explanation: Graphs of linear functions: The blue line, y=12x−3 y = 1 2 x − 3 and the red line, y=−x+5 y = − x + 5 are both linear functions.

Inequalities are arguably used more often in “real life” than equalities. Businesses use inequalities to control inventory, plan production lines, produce pricing models, and for shipping/warehousing goods and materials.

A system of linear inequalities is often used to determine the best solution to a problem. This solution could be as simple as determining how many of a product should be produced to maximize a profit or as complicated as determining the correct combination of drugs to give a patient.

Careers using linear equations range from health care workers to store clerks and everything in between.

• Business Manager.
• Financial Analyst.
• Computer Programmer.
• Research Scientist.
• Professional Engineer.
• Resource Manager.
• Architect and Builder.
• Health Care Professional.

A system of linear inequalities is often used to determine the maximum or minimum values of a situation with multiple constraints. For example, you might be determining how many of a product should be produced to maximize a profit.

The solution of a linear inequality is the ordered pair that is a solution to all inequalities in the system and the graph of the linear inequality is the graph of all solutions of the system. Graph one line at the time in the same coordinate plane and shade the half-plane that satisfies the inequality.