Boundless Chemistry
Q. How do you calculate STP?
STP most commonly is used when performing calculations on gases, such as gas density is calculated using stp = Volume of Gas*(273/Temperature of Gas)*(Pressure of Gas/100). To calculate STP, you need Volume of Gas (V), Temperature of Gas (T) and Pressure of Gas (P).
Table of Contents
- Q. How do you calculate STP?
- Q. What volume would 9.23 moles of oxygen gas occupy at 825 mmHg and 45 C?
- Q. How do you calculate the volume of a gas?
- Q. How many liters of gas are needed for 1 mol?
- Q. What units are gas densities typically given in?
- Q. How do you calculate moles of gas?
- Q. How do you find the moles of a gas given temperature and pressure?
- Q. What is the volume of 0.5 moles of gas at STP?
- Q. What is the volume of 1 moles of gas at STP?
- Q. Which gas occupies the highest volume at STP?
- Q. What is the volume of 2.8 moles of no2 gas at STP?
- Q. What is the volume of 1.5 moles of oxygen gas at standard temperature and pressure STP?
- Q. Why does 1 mole of any gas at STP fill up 22.4 L of volume?
- Q. What is the standard pressure for 1.00 mole of gas at STP?
- Q. What is the value of normal temperature and pressure?
- Q. What is gas law STP?
- Q. Under which conditions of temperature and pressure would helium behave most like an ideal gas?
- Q. Under which conditions of temperature and pressure would a 1 liter sample of a real gas?
- Q. Under which conditions of temperature and pressure does a real gas?
- Q. Under which conditions does a real gas behave?
- Q. Which gas will behave most ideally?
- Q. Why are gases not ideal?
- Q. Why do real gases not always behave ideally?
- Q. Why do gases not behave ideally at low temperatures?
- Q. Can a real gas be condensed?
Q. What volume would 9.23 moles of oxygen gas occupy at 825 mmHg and 45 C?
Answer: Explain:- 22.4L Imol Page 10 Many gas law problems involve calculating the volume of a gas produced by the reaction of volumes of other gases.
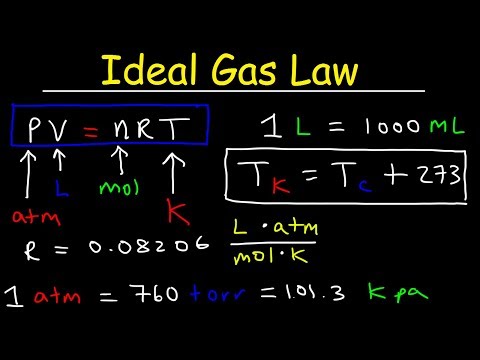
- The ideal gas equation is given by PV=nRT P V = n R T .
- PV=nRT.
- 8.3145L⋅kPaK⋅mol=0.0821L⋅atmK⋅mol=62.4L⋅mm HgK⋅mol.
Q. How do you calculate the volume of a gas?
It can be written as: V = nRT/P. “P” is pressure, “V” is volume, n is the number of moles of a gas, “R” is the molar gas constant and “T” is temperature.
Q. How many liters of gas are needed for 1 mol?
22.4L
Q. What units are gas densities typically given in?
Gas density is defined as the mass of the gas occupying a certain volume at specified pressure and temperature. The density is usually represented in units of lbm/ft3. Another common density representation is the “gas gradient” that is expressed in units of psi/ft.
Q. How do you calculate moles of gas?
Multiply the volume and pressure and divide the product by the temperature and the molar gas constant to calculate moles of the hydrogen gas. In the example, the amount of hydrogen is 202,650 x 0.025 / 293.15 x 8.314472 = 2.078 moles.
Q. How do you find the moles of a gas given temperature and pressure?
The equations describing these laws are special cases of the ideal gas law, PV = nRT, where P is the pressure of the gas, V is its volume, n is the number of moles of the gas, T is its kelvin temperature, and R is the ideal (universal) gas constant.
Q. What is the volume of 0.5 moles of gas at STP?
11.2 L
Q. What is the volume of 1 moles of gas at STP?
22.41 L
Q. Which gas occupies the highest volume at STP?
Argon
Q. What is the volume of 2.8 moles of no2 gas at STP?
Answer. Explanation: 22.4 liters pero bat wala sa mga choices..
Q. What is the volume of 1.5 moles of oxygen gas at standard temperature and pressure STP?
Answer. Answer: The volume occupied is 33.6 Liters. Explanation: STP conditions are known as standard temperature and pressure.
Q. Why does 1 mole of any gas at STP fill up 22.4 L of volume?
The molar volume of a gas is the volume of one mole of a gas at STP. Avogadro’s hypothesis states that equal volumes of any gas at the same temperature and pressure contain the same number of particles. At standard temperature and pressure, 1 mole of any gas occupies 22.4 L.
Q. What is the standard pressure for 1.00 mole of gas at STP?
Standard Pressure is 1 Atm, 101.3kPa or 760 mmHg or torr. At STP, 1 mole of any gas occupies 22.4L.
Q. What is the value of normal temperature and pressure?
A gas industry reference base, and may vary from country to country. Normal, or ambient temperature is 70 °F (21° C). Normal pressure is one atmosphere (1013 hPa) or 14,696 psia.
Q. What is gas law STP?
Standard temperature and pressure (STP) is defined as exactly 100 kPa of pressure (0.986 atm) and 273 K (0°C). This makes for a very useful approximation: any gas at STP has a volume of 22.4 L per mole of gas; that is, the molar volume at STP is 22.4 L/mol (Figure 6.3 “Molar Volume”).
Q. Under which conditions of temperature and pressure would helium behave most like an ideal gas?
Hence, at 750 K and 20 kPa conditions of temperature and pressure does a sample of helium behave most like an ideal gas.
Q. Under which conditions of temperature and pressure would a 1 liter sample of a real gas?
The ideal gas works properly when the inter-molecular interactions between the gas molecules and volume of gas molecule will be negligible. This is possible when pressure is low and temperature is high. Therefore, the correct option is (3) 500 K and 0.1 atm.
Q. Under which conditions of temperature and pressure does a real gas?
In summary, a real gas deviates most from an ideal gas at low temperatures and high pressures. Gases are most ideal at high temperature and low pressure.
Q. Under which conditions does a real gas behave?
Real gas behaves like ideal gas at high temperature and low pressure.
Q. Which gas will behave most ideally?
helium
Q. Why are gases not ideal?
At relatively low pressures, gas molecules have practically no attraction for one another because they are (on average) so far apart, and they behave almost like particles of an ideal gas. At higher pressures, however, the force of attraction is also no longer insignificant.
Q. Why do real gases not always behave ideally?
Why do real gases behave so differently from ideal gases at high pressures and low temperatures? Because the molecules of an ideal gas are assumed to have zero volume, the volume available to them for motion is always the same as the volume of the container.
Q. Why do gases not behave ideally at low temperatures?
They never show ideal behavior because of inter-molecular forces (but less stronger than the forces at fluid and at solid states). These forces increase at low temperature because the movement of molecules decrease at these conditions, and the distances between the molecules decrease under high pressure.
Q. Can a real gas be condensed?
Gases are unlike other states of matter in that a gas expands to fill the shape and volume of its container. For this reason, gases can also be compressed so that a relatively large amount of gas can be forced into a small container.