Note that the domain and range are always written from smaller to larger values, or from left to right for domain, and from the bottom of the graph to the top of the graph for range.

To limit the domain or range (x or y values of a graph), you can add the restriction to the end of your equation in curly brackets {}. For example, y=2x{1

Note that the domain and range are always written from smaller to larger values, or from left to right for domain, and from the bottom of the graph to the top of the graph for range. Find the domain and range of the function f whose graph is shown in Figure 1.2. 8.

Example 2: The domain is the set of x -coordinates, {0,1,2} , and the range is the set of y -coordinates, {7,8,9,10} . Note that the domain elements 1 and 2 are associated with more than one range elements, so this is not a function.

The domain is the set of inputs or x-coordinates. The range is the set of outputs of y-coordinates. When both the independent quantity (input) and the dependent quantity (output) are real numbers, a function can be represented by a graph in the coordinate plane.

The range is the difference between the smallest and highest numbers in a list or set. To find the range, first put all the numbers in order. Then subtract (take away) the lowest number from the highest. The answer gives you the range of the list.

Definition of the domain and range The domain is all x-values or inputs of a function and the range is all y-values or outputs of a function. When looking at a graph, the domain is all the values of the graph from left to right. The range is all the values of the graph from down to up.

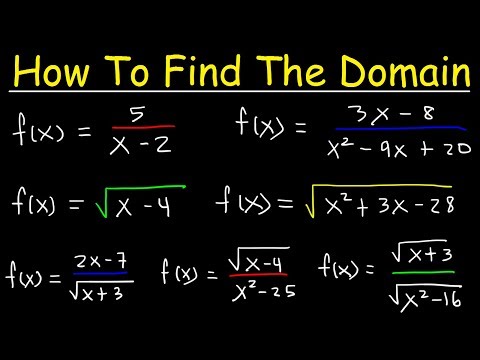
The domain of a function is the complete set of possible values of the independent variable. In plain English, this definition means: The domain is the set of all possible x-values which will make the function “work”, and will output real y-values.

For the quadratic function f(x)=x2 f ( x ) = x 2 , the domain is all real numbers since the horizontal extent of the graph is the whole real number line. Because the graph does not include any negative values for the range, the range is only nonnegative real numbers.

Answer: To find the domain we need to determine the x-values on the graph. If we visualize that the parabola gets infinitely wider from left to right, we can see that the graph will go to negative infinity to the left and to positive infinity on the right.

Negative values can be used for x. The correct answer is: The domain is all real numbers and the range is all real numbers f(x) such that f(x) ≥ 7. C) The domain is all real numbers x such that x ≥ 0 and the range is all real numbers. Negative values can be used for x, but the range is restricted because x2 ≥ 0.

The domain of a function is the complete set of possible values of the independent variable. The domain is the set of all possible x-values which will make the function “work”, and will output real y-values. When finding the domain, remember: The denominator (bottom) of a fraction cannot be zero.

You could set up the relation as a table of ordered pairs. Then, test to see if each element in the domain is matched with exactly one element in the range. If so, you have a function! Watch this tutorial to see how you can determine if a relation is a function.

Any number that can be put on a number line is a real number. Integers like −2, rational numbers/decimals like 0.5, and irrational numbers like √2 or π can all be plotted on the number line, so they are real.

As we saw with integers, the real numbers can be divided into three subsets: negative real numbers, zero, and positive real numbers. Each subset includes fractions, decimals, and irrational numbers according to their algebraic sign (+ or –). Zero is considered neither positive nor negative.

0

The real numbers include natural numbers or counting numbers, whole numbers, integers, rational numbers (fractions and repeating or terminating decimals), and irrational numbers. The set of real numbers is all the numbers that have a location on the number line.