Q. What does zero net change indicate math?
The net change theorem states that when a quantity changes, the final value equals the initial value plus the integral of the rate of change. Net change can be a positive number, a negative number, or zero. For an odd function, the integral over a symmetric interval equals zero, because half the area is negative.
Q. What is mean change?
Mean change is a term used to describe the average change over an entire data set. The mean change is useful for comparing the results of an entire data set to see how the group performed as a whole over a period of time.
Table of Contents
Q. What does total change mean?
The Evaluation Theorem says that if f is continuous on [a,b], then where F is any antiderivative of f. This means that F’=f and equation can be rewritten as .
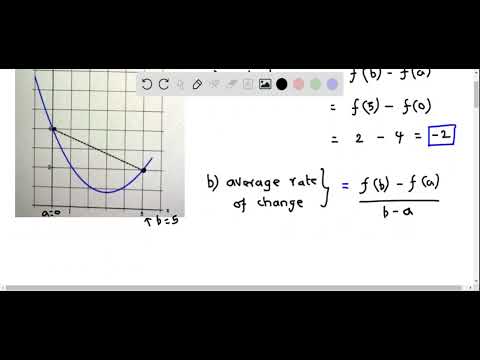
Q. How do you express a slope as a rate of change?
The correct answer is the vertical change divided by the horizontal change between two points on a line. We can find the slope of a line on a graph by counting off the rise and the run between two points. If a line rises 4 units for every 1 unit that it runs, the slope is 4 divided by 1, or 4.
Q. How do I calculate net change?
What is Net Change Formula?
- Net Change Formula = Current Period’s Closing Price – Previous Period’s Closing Price.
- Net Change (%) = [(Current Period’s Closing Price – Previous Period’s Closing Price) / Previous Period’s Closing Price] * 100.
Q. What is a net change in math?
A net change in math is the total of all of the changes completed throughout the solving of a problem. The net change is reflected in a numerical amount and can be positive, negative or at zero. An example of net change can be seen in the equation: X – 5 + 2 = 4.
Q. What is the net change of a function?
In other words, the net change in a function is the (definite) integral of its derivative. In particular, the net distance traveled (final position minus initial position) is the integral of velocity. The net change in velocity (final velocity minus initial velocity) is the integral of acceleration.