Q. What is mass ratio in rockets?
The mass of a vehicle (usually a rocket) at lift-off, divided by the mass of the empty vehicle without propellants. Mass ratio is often used as an indicator of a rocket’s performance. The greater the mass ratio, the more propellant the rocket can carry, resulting in a better lifting performance from the vehicle.
Q. What mass ratio is required for a rocket to leave the Earth’s gravity?
In reality, rockets are multistage, and have typical mass ratios (to Earth escape velocity) of 50 150. For ex- ample, the Saturn V had a total weight of 3,050t for a lunar payload of 45t, so that the ratio is 68.
Table of Contents
- Q. What is mass ratio in rockets?
- Q. What mass ratio is required for a rocket to leave the Earth’s gravity?
- Q. How does the mass of a rocket affect its motion?
- Q. How is a rocket propelled in space?
- Q. What is the ideal mass fraction for a rocket?
- Q. What is inert mass fraction of rocket?
- Q. What is dry weight of rocket?
- Q. How does mass affect acceleration in space?
- Q. How does weight affect the rocket?
- Q. What is the mass ratio of a rocket?
- Q. What is the mass ratio of a Space Shuttle?
- Q. What is the formula for the ideal rocket equation?
- Q. How to calculate the change in momentum of a rocket?
Q. How does the mass of a rocket affect its motion?
Vertical rocket motion A rocket with more mass will speed up more slowly, just as in the horizontal example, but there is another effect. The force of gravity is now acting in the opposite direction to the thrust, so the resultant force pushing the rocket upwards is also less.
Q. How is a rocket propelled in space?
In space, rockets zoom around with no air to push against. Rockets and engines in space behave according to Isaac Newton’s third law of motion: Every action produces an equal and opposite reaction. When a rocket shoots fuel out one end, this propels the rocket forward — no air is required.
Q. What is the ideal mass fraction for a rocket?
Aircraft mass fractions are typically around 0.5. When applied to a rocket as a whole, a low mass fraction is desirable, since it indicates a greater capability for the rocket to deliver payload to orbit for a given amount of fuel.
Q. What is inert mass fraction of rocket?
Inert mass is used because while we know the payload mass, we generally have no way of knowing the structural mass. To find the “inert mass fraction”, divide the inert mass by the total mass of the stage. In existing chemical rocket designs, the inert-mass fraction of a given stage tends to be between 0.08 and 0.7.
Q. What is dry weight of rocket?
This is the average weight of a model rocket by difficulty level:
| Kit Level | Average Dry Weight | Average Motor Weight |
|---|---|---|
| Level 1 | 1.99 oz. / 55.26 g. | 0.64 oz. / 18.20 g. |
| Level 2 | 2.15 oz. / 60.82 g. | 0.79 oz. / 22.37 g. |
| Level 3 | 5.66 oz. / 160.511 g. | 1.60 oz. / 45.26 g. |
| Level 4 | 6.92 oz. / 196.10 g. | 1.73 oz. / 48.97 g. |
Q. How does mass affect acceleration in space?
“What are the factors that affect the acceleration due to gravity?” Mass does not affect the acceleration due to gravity in any measurable way. The two quantities are independent of one another. Light objects accelerate more slowly than heavy objects only when forces other than gravity are also at work.
Q. How does weight affect the rocket?
With any rocket, and especially with liquid-propellant rockets, weight is an important factor. In general, the heavier the rocket, the more the thrust needed to get it off the ground. Because of the pumps and fuel lines, liquid engines are much heavier than solid engines.
Q. What is the mass ratio of a rocket?
Mass ratio. Astrodynamics. Orbital mechanics. In aerospace engineering, mass ratio is a measure of the efficiency of a rocket. It describes how much more massive the vehicle is with propellant than without; that is, the ratio of the rocket’s wet mass (vehicle plus contents plus propellant) to its dry mass (vehicle plus contents).
Q. What is the mass ratio of a Space Shuttle?
The Space Shuttle, for example, has a mass ratio around 16. The definition arises naturally from Tsiolkovsky’s rocket equation : This equation can be rewritten in the following equivalent form:
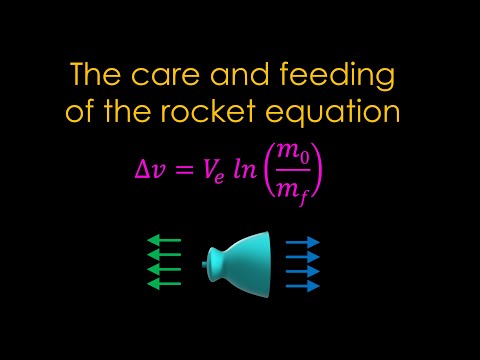
Q. What is the formula for the ideal rocket equation?
delta u = Veq ln (mf / me) This equation is called the ideal rocket equation. There are several additional forms of this equation which we list here: Using the definition of the propellant mass ratio MR MR = mf / me
Q. How to calculate the change in momentum of a rocket?
This changes the mass of the rocket and the velocity of the rocket and we can evaluate the change in momentum of the rocket as. change in rocket momentum = M (u + du) – M u = M du. We can also determine the change in momentum of the small mass dm that is exhausted at velocity v as.