Q. What is Swerling in radar?
Swerling I describes the case in which the target’s velocity is low compared to the observation time, and can thus be considered non-moving. This is the case for a scanning radar, which sweeps its signal past the target in a relatively short time, often on the order of milliseconds.
Q. What is a Swerling 1 target?
Swerling I Target This case describes a target whose magnitude of the backscattered signal is relatively constant during the dwell time. It varies according to a Chi-square probability density function with two degrees of freedom (m = 1).
Table of Contents
- Q. What is Swerling in radar?
- Q. What is a Swerling 1 target?
- Q. What do you mean by RCS fluctuations?
- Q. What is the radar equation?
- Q. How can we reduce RCS?
- Q. What can radar not detect?
- Q. How can I reduce my radar signature?
- Q. What is DB sm?
- Q. How are radar and target models in Swerling?
- Q. What did Peter Swerling contribute to radar research?
- Q. When was the Swerling target model first used?
- Q. What’s the difference between Swerling 1 and 2?
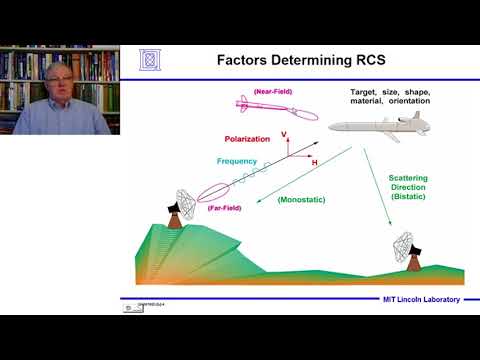
Q. What do you mean by RCS fluctuations?
Radar cross section (RCS) fluctuation characteristics are used to predict radar’s detection performance and evaluate aircraft’s scattering characteristic.
Q. What is the radar equation?
A radar equation relates the range of a radar to the characteristics of the transmitter, receiver, antenna, target, and distance. Assume the radar antenna has an effective area Ae, which is related to the antenna gain by Ae = Grλ2/4π.
Q. How can we reduce RCS?
If the RCS was directly related to the target’s cross-sectional area, the only way to reduce it would be to make the physical profile smaller. Rather, by reflecting much of the radiation away or by absorbing it, the target achieves a smaller radar cross section.
Q. What can radar not detect?
The radar will not work when the received signal power from the object to be detected is too low. This can be due to many different factors: reflection of the signal, absorption of the signal. For example, if the pulse width is 10ns, the radar cannot detect anything within 1.5m.
Q. How can I reduce my radar signature?
Q. What is DB sm?
DBSM. Diplomate in Behavioral Sleep Medicine.
Q. How are radar and target models in Swerling?
In this example, the radar and target are stationary. In Swerling 1 and Swerling 2 target models, the total RCS arises from many independent small scatterers of approximately equal individual RCS.
Q. What did Peter Swerling contribute to radar research?
The models became known as Swerling Target Models Cases I, II, III, and IV in radar literature. In related work, Swerling made significant contributions to the optimal estimation of orbits of satellites and trajectories of missiles.
Q. When was the Swerling target model first used?
Swerling target models are special cases of the Chi-Square target models with specific degrees of freedom. The Swerling models were introduced in 1954 by the American mathematician Peter Swerling and are used to describe the statistical properties of the radar cross-section of objects with complex formed surface.
Q. What’s the difference between Swerling 1 and 2?
Swerling II Target The Swerling II target is similar to Swerling I, using the same equation, except the RCS values changes faster and varies from pulse to pulse additionally. The Swerling cases I and II applies to a target that is made up of many independent scatterers of roughly equal areas like airplanes.