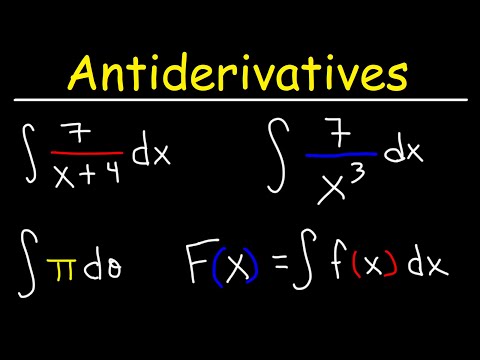
Q. What is the Antiderivative of a derivative?
An antiderivative of a function f(x) is a function whose derivative is equal to f(x). That is, if F′(x)=f(x), then F(x) is an antiderivative of f(x).
Q. What is the relationship between derivatives and integrals?
The derivative and integral are linked in that they are both defined via the concept of the limit: they are inverse operations of each other (a fact sometimes known as the fundamental theorem of calculus): and they are both fundamental to much of modern science as we know it.
Q. What does the Antiderivative represent?
In calculus, an antiderivative, inverse derivative, primitive function, primitive integral or indefinite integral of a function f is a differentiable function F whose derivative is equal to the original function f. This can be stated symbolically as F’ = f.
Q. Are integrals and Antiderivatives the same thing?
The answer that I have always seen: An integral usually has a defined limit where as an antiderivative is usually a general case and will most always have a +C, the constant of integration, at the end of it. This is the only difference between the two other than that they are completely the same.
Q. Can a function have two Antiderivatives?
Thus any two antiderivative of the same function on any interval, can differ only by a constant. The antiderivative is therefore not unique, but is “unique up to a constant”. The square root of 4 is not unique; but it is unique up to a sign: we can write it as 2.
Q. What is the first and second fundamental theorem of calculus?
Formal statements. There are two parts to the theorem. The first part deals with the derivative of an antiderivative, while the second part deals with the relationship between antiderivatives and definite integrals.
Q. How do you use the first fundamental theorem of calculus?
The First Fundamental Theorem of Calculus. Let f(x) be a continuous positive function between a and b and consider the region below the curve y = f(x), above the x-axis and between the vertical lines x = a and x = b as in the picture below. and call this the definite integral of f(x) from a to b.
Q. Who first proved the fundamental theorem of calculus?
Sir Isaac Newton
Q. How many fundamental theorems of calculus are there?
There are really two versions of the fundamental theorem of calculus, and we go through the connection here. Created by Sal Khan.
Q. What is the fundamental theorem of calculus used for in real life?
As mentioned earlier, the Fundamental Theorem of Calculus is an extremely powerful theorem that establishes the relationship between differentiation and integration, and gives us a way to evaluate definite integrals without using Riemann sums or calculating areas.
Q. What is the application of limits in real life?
Examples of limits: For instance, measuring the temperature of an ice cube sunk in a warm glass of water is a limit. Other examples, like measuring the strength of an electric, magnetic or gravitational field. The real life limits are used any time, a real world application approaches a steady solution.
Q. How do you introduce the concept of limits?
Using correct notation, describe the limit of a function. Use a table of values to estimate the limit of a function or to identify when the limit does not exist. Use a graph to estimate the limit of a function or to identify when the limit does not exist.
Q. How do you represent limits?
The symbol lim means we’re taking a limit of something. The expression to the right of lim is the expression we’re taking the limit of. In our case, that’s the function f. The expression x → 3 x/to 3 x→3 that comes below lim means that we take the limit of f as values of x approach 3.
Q. Why are limits so hard to understand?
A big problem with understanding the limit definition, as you have pointed out, is the problem of quantifiers. There are so many, and the order in which they are employed is so important, that it can be difficult to keep track of. A big problem with using the limit definition, is that it feels circular.
