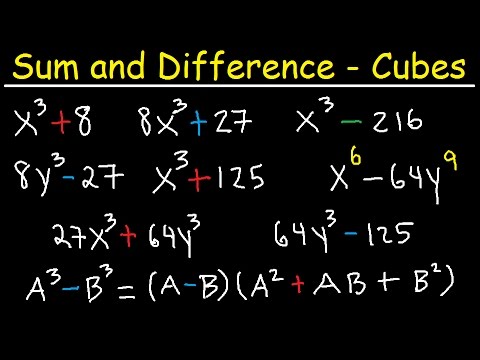
Q. What is the example of factoring sum and difference of two cubes?
The sum or difference of two cubes can be factored into a product of a binomial times a trinomial. That is, x3+y3=(x+y)(x2−xy+y2) and x3−y3=(x−y)(x2+xy+y2) .
Q. What is the example of sum of two cubes?
Examples on Sum of Cubes Formula To find: Factor of 216×3 + 64, using the sum of cubes formula. Answer: The factor of 216×3 + 64 is 2(3x + 2)(9×2 – 6x + 4). Example 2: Find the factor of 8×3 + 125y3. To find: Factor of 8×3 + 125y3, using the sum of cubes formula.
Q. What is a difference of two cubes?
The difference of two cubes is equal to the difference of their cube roots times a trinomial, which contains the squares of the cube roots and the opposite of the product of the cube roots.
Q. What is the difference of two cubes?
The difference of two cubes is equal to the difference of their cube roots times a trinomial, which contains the squares of the cube roots and the opposite of the product of the cube roots. A number’s opposite is that same number with a different sign in front.
Q. How do you factor a cubed expression?
The factored form of a3 + b3 is (a + b)(a2 – ab + b2): (a + b)(a2 – ab + b2) = a3 + a2b – a2b – ab2 + ab2 + b3 = a3 – b3. For example, the factored form of 64×3 + 125 (a = 4x, b = 5) is (4x + 5)(16×2 – 20x + 25).
Q. How to factor the difference of two cubes?
Apply the rule for difference of two cubes, and simplify. Since this is the “difference” case, the binomial factor and trinomial factor will have negative and positive middle signs, respectively. 27 {x^3} + 64 {y^3} 27×3 + 64y3. The first step as always is to express each term as cubes.
Q. Which is the greatest common factor of two cubes?
For the numbers, the greatest common factor is xy xy “. Therefore the overall common factor would be their product which is //left ( 3 ight)//left ( {xy} ight) = 3xy (3) (xy) = 3xy. After factoring it out, you’ll see that we have an easy problem on the difference of two cubes.
Q. How to factor out the negative in sum of cubes?
Direct link to Sahil Acharya’s post “They would factor out the…” They would factor out the negative: – (a^3+b^3), then factor the difference of cubes, which follows almost exactly the same format as sum of cubes. Sum of cubes is (a-b) (a^2-ab+b^2). Note the negative sign between a^3 and ab
Q. Which is Sal factor for difference of cubes?
Sal factors 40c^3-5d^3 as 5 (2x-d) (4c^2+2cd+d^2) using a special product form for a difference of cubes. Created by Sal Khan.
